Interest Calculator
| Ending balance | $0.00 |
| Total principal | $0.00 |
| Total contributions | $0.00 |
| Total interest | $0.00 |
| Interest of initial investment | $0.00 |
| Interest of the contributions | $0.00 |
| Buying power of the end balance after inflation adjustment |
$0.00 |
Future Value (FV) =
P × (1 + r/n)nt + PMT × [((1 + r/n)nt - 1) / (r/n)] × (1 + r/n) (if contribution at beginning)
or
P × (1 + r/n)nt + PMT × [((1 + r/n)nt - 1) / (r/n)] (if contribution at end)
Where:
P = Initial investment
PMT = Contribution per compounding period
r = Annual interest rate (decimal)
n = Compounding frequency per year
t = Time in years
Example:
For P = $10,000, PMT = $500 (per period), r = 0.05 (5%), n = 12 (monthly), t = 5 years:
Future Value = $10,000 × (1 + 0.05/12)12×5 + $500 × [((1 + 0.05/12)12×5 - 1) / (0.05/12)]
Results:
Future Value = $48,267.34
Total Interest = $7,767.34
Buying Power after 2% Inflation = $43,732.12
What is Interest?
Interest is the cost of borrowing money or the reward for saving it. It’s usually shown as a percentage of the original amount, known as the principal. Interest is at the heart of most financial products, from loans and mortgages to savings accounts and investments.
There are two main types of interest: simple interest and compound interest. Understanding the difference can help you make smarter financial decisions.
Simple Interest Explained
Let’s break down a simple example. Say Derek borrows $100 from a bank for one year at a 10% interest rate.
$100 × 10% = $10
After one year, Derek owes:
$100 (principal) + $10 (interest) = $110
If the loan lasts two years and the interest is calculated annually without compounding:
Year 1: $10 interest
Year 2: $10 interest
Total repayment: $120
Simple Interest Formula:
Interest = Principal × Rate × Time
If interest is calculated more frequently (monthly or daily), the formula adjusts to include the number of periods:
Interest = Principal × Rate × Time ÷ Frequency
However, simple interest is rarely used in modern finance. Most interest in banking and investing is compound interest.
Understanding Compound Interest
Compound interest means you earn interest not only on the principal but also on previously earned interest. Let’s go back to Derek’s example.
Year 1: $100 × 10% = $10 → Total: $110
Year 2: $110 × 10% = $11 → Total: $121
With compound interest, Derek pays $121 instead of $120 under simple interest. Over time, this difference grows bigger, especially with frequent compounding.
The more often interest is compounded, the more your money grows. Here’s how a $1,000 investment with 20% interest performs at different compounding frequencies:
Annually → Less growth
Monthly, Daily → More growth
Continuously → Maximum growth
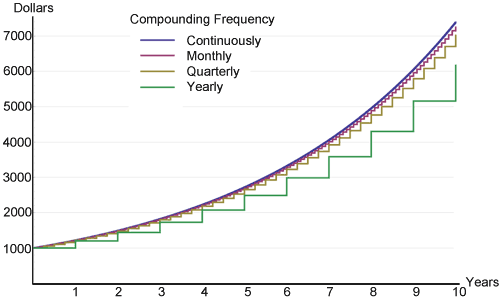
This is the power of compound interest, and it’s why it’s often called “interest on interest.”
The Rule of 72
Want a quick way to estimate how long it takes to double your money? Use the Rule of 72:
Years to double = 72 ÷ Interest Rate
Example: At 8% interest, it takes 72 ÷ 8 = 9 years to double your money.
This shortcut works best with rates between 6–10%, but it’s a helpful trick even outside that range.
Fixed vs. Floating Interest Rates
Interest rates come in two types:
Fixed rate – stays the same for the loan or investment term.
Floating rate – changes based on benchmarks like the Federal Reserve rate or LIBOR.
For example, a floating rate loan may rise if market rates go up. On the other hand, fixed-rate loans offer predictability, which is what this calculator is designed to handle.
Adding Regular Contributions
Our Interest Calculator also lets you include recurring contributions (like monthly savings). One key detail: adding money at the beginning of each compounding period earns more interest than adding at the end.
This feature helps model real-world savings plans like retirement contributions or college funds.
How Taxes Affect Interest Earnings
In many countries, including the U.S., certain types of interest income are taxable. For example:
Corporate bonds – typically fully taxed
Federal bonds – taxed federally but may be exempt from state taxes
Let’s see how this affects savings. If Derek saves $100 at 6% for 20 years, his balance without taxes:
$100 × (1 + 6%)²⁰ = $320.71
But if taxed at 25%, he only ends up with:
$239.78 – a big difference!
Always consider tax implications when calculating interest.
Don’t Forget About Inflation
Inflation reduces your money’s buying power over time. If inflation averages 3% annually, your savings need to grow faster than that just to keep their real value.
For context, the U.S. stock market (S&P 500) has returned about 10% on average over the last century, while inflation hovered around 3%. That’s why your real rate of return matters.
Tip: Set the inflation rate to 0 for basic calculations, but include it for more accurate results using our calculator.
Real Interest Rate = Nominal Rate – Inflation – Taxes
To truly grow your wealth, your interest earnings must outpace both inflation and taxes. For example, with a 25% tax rate and 3% inflation, you’d need at least 4%+ in returns to maintain your purchasing power.
Financial Calculators
- Mortgage
- Amortization
- Mortgage Payoff
- House Affordability
- Rent
- Debt-to-Income Ratio
- Real Estate
- Refinance
- Rental Property
- APR
- FHA Loan
- VA Mortgage
- Down Payment
- Rent vs. Buy
- Loan
- Payment
- Currency
- Inflation
- Sales Tax
- Credit Card
- Credit Cards Payoff
- Debt Payoff
- Debt Consolidation
- Repayment
- Student Loan
- College Cost
- VAT
- Depriciation
- Margin
- Discount
- Business Loan
- Boat Loan
- Personal Loan
- Lease
- Budget
- Commission
- Investment
- Finance
- Compound Interest
- Interest Rate
- Savings
- Simple Interest
- CD
- Bond
- Average Return
- IRR
- ROI
- Payback Period
- Present Value
- Future Value
- Income Tax
- Salary
- Marriage Tax
- Estate Tax
- Take-Home-Paycheck
- Auto Loan
- Cash Back or Low Interest
- Auto Lease
- Retirement
- 401K
- Pension
- Social Security
- Annuity
- Annuity Payout
- Roth IRA
- IRA
- RMD
